Kylerec – Seiberg-Witten and Fillings

The first post on Kylerec is here, so if you haven’t been keeping up with these posts, you might want to start there. This is also the last post on the Kylerec 2017 workshop, which has been fun and rewarding to write about (with some much appreciated help from Agustín Moreno)!

If you’re following along with the lecture notes from Kylerec, then this post corresponds to Day 4 (Talks 11-14), consisting of the following four talks:

• My talk following sections 2-3 of Hutchings and Taubes Introduction to the Seiberg-Witten equations.
• Jie Min’s talk, on section 4 of those notes (the symplectic manifold case), but also continuing on to Morgan and Szabó’s paper which can be used to classify homotopy K3 surfaces
• Tom Gannon’s talk on fillings of unit contangent bundles, following Li-Mak-Yasui and Sivek–Van Horn-Morris
• Dani Álvarez-Gavela’s talk on how Lisca and Matić distinguish contact structures using Seiberg-Witten theory

 

A pre-introduction to Seiberg-Witten theory

The Seiberg-Witten equations have been discussed in this blog by Laura Starkston in a sequence of four posts. For more information, details, and clarification, the interested reader should go there, or to the notes of Hutchings and Taubes mentioned in the introduction to this post. I call this a pre-introduction because the details will be rather sketchy. I will not even write the Seiberg-Witten equations down. The reader interested in skipping to fillings may wish to jump ahead to the two-sentence summary at the end of this section.

I should mention that the Seiberg-Witten equations arise naturally in physics, although I’ve not yet personally taken the time to understand Witten’s motivation for first writing down these equations, which he called the monopole equations. If you are interested in that sort of thing, maybe check out this MathOverflow post.

Consider a closed oriented smooth 4-manifold , together with the following data:

• a Riemannian metric
• a self-dual 2-form (meaning where is the Hodge star with respect to )
• a -structure

You might be asking – what’s a -structure? Recall that for , one has , so one can form the connected double cover . Then one defines the Lie group

This comes with a map to with fiber . The metric yields a principal -bundle called the frame bundle, which topologically doesn’t depend on the metric, and a -structure is just a principal -bundle such that quotienting by the -action (sitting inside the -action) recovers the frame bundle.

For oriented, which is the case of interest to us, the space of -structures, , is an affine space modelled on (this is not obvious). Also in the 4-dimensional case, representation theory of the Lie group yields two complex 2-dimensional spinor bundles and a complex line bundle . The Seiberg-Witten equations are then equations on pairs consisting of a -connection on and a positive spinor (a section of ). We write this simply as

.

Let be the solutions to this eqution. There is an action of the gauge group (given by ). This action is free except for reducible solutions where , in which case the stabilizer is . The quotient yields the moduli space (where we suppress from the notation):

Theorem (key, nontrivial): The space is always compact.

Let be the rank of the positive-definite part of with respect to the intersection product. We will assume . This implies that generically paths of choices for a fixed will avoid reducible solutions, yielding the following.

Theorem (standard Fredholm theory): Consider with and some fixed . Then generically (with respect to ),

• is a smooth finite-dimensional manifold of dimension given by topological data (only depending on and )
• can be given an orientation with some auxiliary topological choice (not depending on )
• is a cobordism invariant

Definition: For , and for with , we define the Seiberg-Witten invariant , where we count with signs according to the auxiliary topological choice.

One can also define the Seiberg-Witten invariant when the dimension is positive, but there is the simple type conjecture that in such cases, this invariant is zero. In the case of symplectic manifolds, which is the case we care about, this is known to be true (by Taubes). By construction, the Seiberg-Witten invariants are diffeomorphism invariants (once we have fixed our auxiliary data for determining an orientation of ).

We are interested in the case of symplectic manifolds. In this case, there is a canonical choice for the data which orients the moduli spaces of solutions to the Seiberg-Witten equations. There is a natural morphism given by the where is the line bundle mentioned before. (This is not an isomorphism if has 2-torsion.)

Definition: A class is basic if there is a -structure with such that .

We finish by stating the following facts without proof (although we did discuss the proofs at Kylerec).

Theorem [Taubes]: For a symplectic manifold, are basic classes (with Seiberg-Witten invariants ).

Theorem [Corollary of the same Taubes paper]: When is minimal, Kähler, and of general type (the last condition meaning and ), then are the only basic classes.

Theorem [Corollary of Morgan-Szabó]: If has , , and , then it is a rational homology K3 surface.

SUMMARY OF THIS SECTION: The Seiberg-Witten invariants form a diffeomorphism invariant, hence so do basic cohomology classes. This fact, plus the previous three theorems, are all we need.

 

Filling unit cotangent bundles

Unit cotangent bundles, which we shall notate as , have canonical Weinstein fillings given by the unit disk cotangent bundles. It is a natural question to ask if this natural filling is in fact the only one up to some notion of equivalence. We shall restrict ourselves in this discussion to when the base space is a closed orientable surface of genus . We mostly focus on the case, but we quickly review the case of .

Let us begin with . In the first post on J-holomorphic curves, when discussing McDuff’s classification result, I mentioned that has a unique minimal strong filling up to diffeomorphism. Further, Hind proved that Stein fillings are unique up to Stein homotopy.

For , in the second post on J-holomorphic curves, when discussing Wendl’s J-holomorphic foliations, I mentioned that every minimal strong filling of to the standard one. In fact, he proves further that every minimal strong filling is symplectically deformation equivalent, which is a little stronger. Also, Stipsicz proved that all Stein fillings are homeomorphic (to ).

To summarize roughly (though we know a little more), for , exact fillings (which are automatically minimal) are unique up to symplectic deformation equivalence.

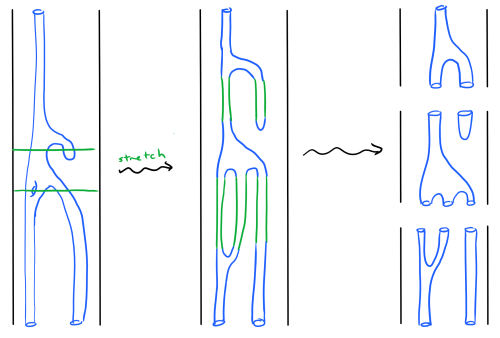
So now we move on to . We focus on exact fillings because strong fillings (even minimal ones) are too weak to get a handle on. One can build strong fillings with arbitrarily large positive second Betti number . This involves cutting out a cap (with concave boundary) from one particular strong filling (McDuff) and gluing in other caps with higher (Etnyre and Honda).

The idea, in this paper of Li, Mak, and Yasui, is similar to the idea we encountered in McDuff’s approach to the (and more generally ) case – attach a cap, and then use classification results to figure out what you had in the first place. The following definition is the correct version of cap that we need.

Definition: A Calabi-Yau cap for a contact 3-manifold is a strong cap (like a filling, but with a concave end instead) with torsion.

Theorem 1 [LMY]: If a Calabi-Yau cap exists for , then the set of triples of Betti numbers is finite as ranges over all exact fillings.

Remark: This theorem is not true if instead we let range over all strong fillings. This was noted above when we remarked that could be arbitrarily large for a strong filling.

Theorem 2 [LMY]: In the case of the unit cotangent bundle, then for any exact filling , its homology and intersection form are the same as that of the standard filling.

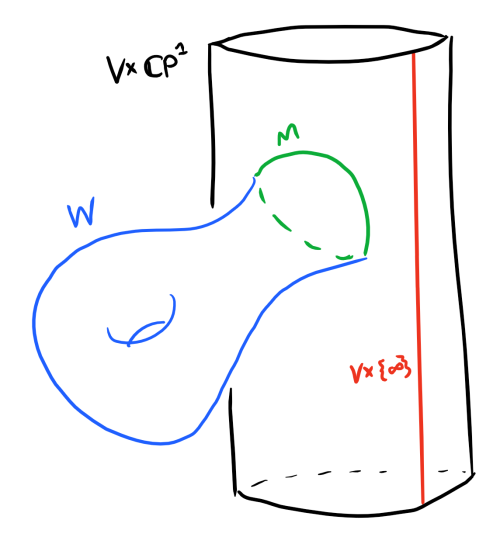
Sketch Proof of Theorem 1: Some messing around with Chern classes tells us that if we have an exact filling and a Calabi-Yau cap for , then the glued manifold satisfies . Then one can plug this into classification theorems by an invariant called the symplectic Kodaira dimension . In the case when is minimal with , we must have . In this case, when , then the Morgan-Szabó result mentioned in the Seiberg-Witten section implies that we have a rational K3 surface, hence we know its Betti numbers. Tian-Jun Li extended this result to a classification for and minimal but with arbitrary . Otherwise, if is not minimal, it must have , and one needs to be a little more careful, working with a symplectic surface in to which an adjunction inequality ends up bounded the Betti numbers.

Sketch Proof of Theorem 2: The key lemma is to construct a symplectic K3 surface with non-intersecting Lagrangian tori in the same homology class which all intersect a Lagrangian sphere transversely in one point. Then we can perform Lagrangian surgery to give an embedded Lagrangian genus surface . Then is a Calabi-Yau cap for . Playing around with intersection forms, we see that attaching this cap must yield a rational K3 surface (one can rule out all other possibilities given by the classification theorems mentioned in the proof of Theorem 1), from which playing around more with exact sequences of homology and intersection forms gives the result.

Remark: The classification-type results with respect to symplectic Kodaira dimension are the only place in this section where Seiberg-Witten equations enter the picture, and are really the meat of the argument, in some sense. The rest just comes from exact sequences and understanding intersection forms, which is comparatively simple, staying far away from gauge theory.

The main theorem of Sivek and Van Horn-Morris is the following:

Theorem [SV]: Weinstein fillings of are unique up to s-cobordism rel boundary.

If you’re worried about the word “s-cobordism,” just think of this as a beefed up version of homotopy equivalence that comes relatively easily in this case once we prove the homotopy type of the filling is unique (is a ). There are some beautiful group-theoretic arguments which go into this argument, but we have essentially already seen how the Seiberg-Witten invariants come into play, so I won’t include a sketch of the proof.

Finally, I mention a little bit of history with regards to these two papers, because I was confused looking at the most recent versions as I was writing this, not for lack of improper attributions, just by my own confusions about reading them concurrently. The theorems stated are quite similar, as are aspects of the proofs, despite them being stumbled upon independently. To clarify, Theorem 2 of LMY did not exist in version 1 of their paper. About a year later, within a month of each other, SV posted their paper and LMY posted version 2 of their paper. Independently, SV had proved some subset of Theorem 2 (with some small fudge factor in and the intersection form) while LMY had proved the full version. SV’s result was good enough for them to prove the s-cobordism statement, and as far as I can tell, version 2 of SV is just version 1 but where they mention that they have learned that LMY proved the strong version of Theorem 2.

 

Homotopic tight contact structures which are different

The main theorem, due to Lisca and Matić, is the following:

Theorem [LM]: For any , there exists a rational homology 3-sphere with at least distinct contact structures up to contactomorphism which are homotopic as plane fields.

In this short section, we simply sketch the proof.

Sketch of proof: One must begin by simply writing the Gompf surgery diagrams (described in the post on Weinstein fillings) for the contact structures in question. One has that a rational homology sphere can be obtained by 0-surgery on a trefoil and -surgery on an unknot which links with the trefoil once, and so suggests the following surgery diagrams so that the canonical framing on the Legendrians drawn below gives exactly what we want.

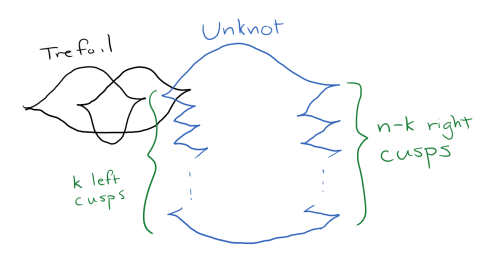
We denote these contact structures by for . We will show that for a fixed , all of these are homotopic but not contactomorphic. One computes via results of Eliashberg that for the corresponding Weinstein fillings (which are diffeomorphic) that , where is the class in given by the handle coming from the trefoil in the surgery. We shall call the smooth underlying manifold .

The homotopic part is rather simple. By classical results (clutching functions, and computing Pontrjagin classes to plug into the Hirzebruch signature theorem) following an argument attributed to Gompf, one can show that the homotopic result can be reduced to proving that , which is itself clear since .

As for the contactomorphism part, one embeds into a minimal compact Kähler surface of general type and . This is a nontrivial statement, but is nonetheless true. In fact, because and have isomorphic collars, one can attach the same cap to produce Kähler surfaces and . One can extend the identity on these caps to an orientation preserving diffeomorphism acting by on (by work of Gompf). But also, since we have a minimal compact Kähler manifold, by the theorem mentioned in the first section as a corollary of Taubes’ work, one has that is a diffeomorphism invariant, and so we see that . So these must restrict to the same thing on , where we showed . Hence, , so either or . Thus, increasing , we can find arbitrarily many homotopic but non-contactomorphic contact structures.

Kylerec – Weinstein fillings

Continuing on with the Kylerec posts… (see the first one here as well as notes to follow along with here).

This post is a synthesis of the following talks:

• Day 1 Talk 2 – François-Simon Fauteux-Chapleau’s talk on Weinstein handles and contact surgery
• Day 1 Talk 3 – Orsola Capovilla-Searle’s talk on Kirby calculus for Stein manifolds
• Day 1 Talk 4 – Alvin Jin’s talk on Lefschetz fibrations and open books
• Day 2 Talk 1 – Bahar Acu’s talk on mapping class factorizations and Lefschetz fibration fillings
• Day 3 Talk 2 – Sarah McConnell’s talk on applications of Wendl’s theorem to fillings
• Day 5 Talk 1 – Ziva Myer’s talk on flexible and loose Legendrians

 

Weinstein surgery theory

I assume the reader is familiar with smooth surgery theory. Recall the following definition.

Definition: A Weinstein cobordism consists of a quadruple , where

• is a compact symplectic manifold with boundary
• is a Liouville vector field for , meaning , which is also transverse to the boundary
• is a Morse function
• is gradient-like for , meaning there is some constant with with respect to a given Riemannian metric.

In this case, the boundary decomposes as , where points out of and into . Note that the 1-form satisfies , and is sometimes called the Liouville 1-form, since it encodes the same data as . Also note that a Weinstein cobordism with is what we called a Weinstein filling.

The gradient-like condition is meant to give some directionality (since ) and ensure that the critical points of are non-degenerate. One typically doesn’t think of the precise choice of pair as very important, but rather the data up to some notion of homotopy. For example, one can always perturb the Morse function so that each of and is a regular -level set, regardless of the number of components, and so we might as well assume this from the start. The equivalence hinted at here is called Weinstein homotopy, by which we perturb the pair , possibly through birth-death type singularities.

Lemma: The descending manifolds in a Weinstein cobordism, i.e. the set of points which flow along to a given critical point in infinite time, are isotropic submanifolds.

Proof: Standard Morse theory implies these submanifolds are smooth. Let be the flow along at time , and suppose we choose some where is some descending manifold for a given critical point . Suppose is a vector in the tangent space. Then since , we have that

As , the right hand side goes to zero since for all in a curve along with tangent vector at . Hence, , from which it follows that . Hence, , and so also .

Corollary: All critical points in a Weinstein cobordism are of index at most . Smoothly, any such manifold can be built up by surgery starting from a neighborhood of and attaching handles of index at most .

One would like to be a bit more precise about how the surgery interacts with the symplectic geometry. As a first step, along a regular level set , the symplectic condition on implies that is a contact form. The proof of the lemma above further implies that gives an isotropic submanifold of with respect to .

So we can think, at least smoothly, that our Weinstein cobordism is built up, starting from , by attaching handles with isotropic cores and attaching spheres along isotropics in level sets of (which are contact submanifolds). But there’s a little more that we know about neighborhoods of isotropics. In a symplectic manifold, the neighborhood of an isotropic is completely determined up to symplectomorphism by its symplectic normal bundle, , as a symplectic vector bundle (with symplectic structure induced by on the fibers).  A similar statement holds for isotropic submanifolds in contact manifolds, but now with their neighborhoods determined up to contactomorphism by the conformal symplectic normal bundle , where is a contact form so that is symplectic on . Furthermore, if we fix , then the symplectic vector bundle structure determined by on the nose determines the neighborhood up to exact contactomorphism. Patching these two things together, one finds:

Theorem [Weinstein, before the term “Weinstein handle” was coined]: Weinstein handle attachment is completely specified (up to Weinstein homotopy) by matching the symplectic framing data determined by along the isotropic attaching spheres.

One therefore thinks of as being built up from by contact surgery along isotropic submanifolds with given framing information compatible with the underlying symplectic topology.

Consider a Weinstein cobordism of dimension . Then the handles of index are called subcritical handles, whereas the handles of index are called critical handles. When , the aformenetioned symplectic normal bundles are trivial automatically, and so one specifies critical handle attachment simply by drawing a Legendrian sphere on .

Recall that the proof of the h-cobordism theorem requires some ability to cancel (and create) pairs of handles with index differing by 1 whose ascending and descending manifolds intersect in a 1-dimensional manifold, to move around attaching spheres, and to move critical values around. The last of these we can always do, so we can attach the handles in order of their index. It turns out that when , we can recreate all parts of the proof of the h-cobordism theorem for subcritical Weinstein cobordisms. In some sense, subcritical Weinstein domains have no symplectic geometry in them – they are encoded by algebro-topological information, and so this gives some flexibility phenomena.

It turns out that some critical handles behave the same way. The key obstruction to the aforementioned flexibility is that sometimes the data of an attaching Legendrian does not boil down to purely toplogical information. However, Emmy Murphy defined a class of Legendrians, called loose Legendrians, for which there is such a so-called h-principle. The Weinstein h-cobordism theorem works for Weinstein cobordisms which can be built (up to Weinstein homotopy) out of subcritical and loose critical handle attachments. We call such Weinstein cobordisms flexible.

We often care about the case when . In this case, it is pretty easy to describe a connected Weinstein domain (or its contact boundary). One can first order the handles by index, and then cancel 0-handles with 1-handles until we are in the situation where there is precisely one 0-handle and possibly many 1- and 2-handles. The boundary of the 0-handle is just a standard contact , and 1-handle attachment is trivially described by picking pairs of points in (the bundle data boils down to showing ). So it suffices to draw Legendrians on with pairs of points identified, which is just . Any Legendrian has a canonical framing of its normal bundle given by the twisting of the Reeb chord around the Legendrian. Eliashberg showed that adding a left twist to this framing gives the smooth framing which determines the corresponding smooth surgery data.

Gompf showed that in this case , one can draw standard Kirby calculus type surgery diagrams. We think of all of these 1-handle attachments and Legendrians as missing a point in , so that we can draw our diagrams in . The front projection is the projection to the coordinates , so that is determined by . It might not be obvious how to draw a smooth knot in this projection since the curve can’t have infinite slope, but we are allowed semi-cubical cusps, corresponding to . Note that transverse crossings are also allowed, since the -coordinates are distinct. One usually draws the front projection of a Legendrian without showing which strand lies over the other, but we include this extra information in the next figure, where we imagine the -axis as pointing into the page.

A Legendrian trefoil knot

Gompf’s standard form for these Legendrians looks like the following, where the pairs of balls in each row corresponds to where the 1-handles are attached, and the Legendrian strands simply go through the handles as though they were wormholes.

An example of a Gompf surgery diagram. There are three 1-handles (in blue, red, and green) and two 2-handles with attaching spheres given by the Legendrian tangle above. All of the information can be made to live inside of the purple rectangle (i.e. without going horizontally or vertically outside of where the 1-handles are attached).

 

Weinstein fillings, Lefschetz fibrations, and open book decompositions

Definition: A Lefschetz fibration is a smooth map with finitely many critical points with distinct critical values such that locally around the critical points, looks like a complex Morse function (i.e. in local coordinates). When has boundary, we assume the critical values of are all in the interior of .

We shall typically be concerned with the case where (although see this post by Laura Starkston which slightly generalizes some of what is discussed here).

A schematic for a Lefschetz fibration over the disk

In the case where , we see that the boundary decomposes as , where the superscripts are meant to indicate vertical and horizontal. That is, , while . If we write for a regular fiber of , then . Meanwhile, we see that is just a fibration over with fiber , and hence can be described by some monodromy map fixing the boundary, so that where (the mapping torus of ).

The structure on the boundary, in which we have a fibration over with fiber glued together with in the natural way, is called an open book decomposition. It is given completely by the pair . We think of each fiber over as a page, and the subset as the binding, analogous to what one would get if one took their favorite book and matched the covers so that the pages radiate outwards. So Lefschetz fibrations yield open books on the boundary. To be a little more precise, one should extend each page so that the boundary of each page is actually the binding.

Some pages near the binding of an open book. I guess the name “Rolodex” wasn’t as catchy as “open book.” (Image from Wikipedia)

Now suppose is a regular value (which can always be arranged up to small perturbation of ). Then . One can ask what happens when we extend to , where and there is exactly one critical value on .

Since we have a nice fibration away from critical points, we see that paths in yield monodromy maps (up to isotopy preserving boundary) on the fibers. We can choose a connection on the fibration if we wish to make this a map on fibers, not just a map up to isotopy. If we take a path from 0 to which intersects once and otherwise avoids critical values then for whatever connection we chose, we can see what points flow to the critical point over . Over each regular fiber, this is just a circle, and the union of all of them together with the critical point yields a disk. The path is called a vanishing path, and each circle on the regular fiber is called a vanishing cycle (one really should think of it as a homology cycle, but for concreteness, one can think of it as a curve). The disk consisting of the union of vanishing cycles above a path is called a thimble.

The green circles in the regular fibers above the purple vanishing path are the vanishing cycles. Their union is the thimble.

It is then not hard to see that is obtained from by 2-handle attachment, where the attaching curve is just the vanishing cycle above and the core of the handle is the thimble. Furthermore, one can check by a local computation that the monodromy map in a loop around is just given by a Dehn twist (positive or negative, depending on orientations) around the vanishing cycle. Hence, one can write out the open book determined by the Lefschetz fibration explicitly – it is just the product of the Dehn twists on the vanishing cycles, performed in an order determined by a sequence of vanishing paths.

Notice that for a given regular value on , one can choose a different basis of vanishing paths, and this yields a possibly different factorization for the monodromy. Such changing of the basis is generated by so-called Hurwitz moves, as drawn below.

A Hurwitz move swapping the th and st critical points. Note that the corresponding vanishing cycles for the critical point corresponding to and are actually different, but the overall monodromy on the open book at the boundary is the same.

Hence, understanding Lefschetz fibrations over the disk essentially corresponds to understanding factorizations of mapping class group elements into Dehn twists.

Now, this whole story can be repeated in the symplectic context, as follows.

Definition: A symplectic Lefschetz fibration is a Lefschetz fibration with a symplectic manifold such that each fiber is symplectic submanifold away from the critical points, while at the critical points the coordinates in which locally looks like a complex Morse function can be taken to be holomorphic for some compatible almost complex structure .

In this case, one can take the connection to be the symplectic connection given the symplectic orthogonal complement to the vertical directions. In this way, the thimbles produced will actually be Lagrangian disks, which suggests one can think of these as the descending disks for a Weinstein domain filling the boundary. In addition, the monodromy maps are now compositions of positive Dehn twists only, since the symplectic condition gives the proper orientations. In other words, our Lefschetz fibration is itself positive. If the vanishing cycles of a Lefschetz fibration are homologically nontrivial, we shall call it allowable.

With a little more work, we can obtain the following theorem of Loi and Piergallini (although an alternative proof by Akbulut and Özbağci is more in line with the exposition presented here):

Theorem: Any positive allowable Lefschetz fibration (PALF) yields a Weinstein domain, and any Weinstein domain comes from a PALF in this way.

Furthermore, one obtains a little bit more compatibility at the boundary.

Definition: An open book decomposition on a manifold is said to support a cooriented contact structure if there is some contact form for such that the binding is a contact submanifold, is a symplectic form on the pages, and the boundary orientation of the page (with respect to ) matches the orientation of the binding with respect to .

One checks that the open book on the boundary of a PALF does indeed support the contact structure determined by being the boundary of a Weinstein domain.

Our surgery theory for these Lefschetz fibration builds the fiber up by subcritical surgery, and the 2-handle attachments correspond to the critical points of the fibration. One can always produce, for any Weinstein manifold, a cancelling pair consisting of a 1-handle and a 2-handle. The way that this affects the open book is by positive stabilization, meaning that one adds a 1-handle to the page, but kills it by adding an extra Dehn twist to the monodromy through a circle which passes through the handle.

The following theorem implies that all 3-dimensional contact geometry can actually be encoded (somewhat non-trivially) in the study of open books up to positive stabilization, and hence the study of Weinstein fillings reduces to studying positive factorizations of given elements of the mapping class group of a surface with boundary (up to this not-so-easy-to-work-with notion of positive stabilization).

Theorem [Giroux correspondence]: There is a one-to-one correspondence between contact structures on a closed 3-manifold up to isotopy with open books up to positive stabilization.

 

Applications to Weinstein fillings

To summarize the previous section, an explicit surgery decomposition of a Weinstein filling yields a PALF which in turn gives an open book structure supporting the contact boundary of the Weinstein filling with monodromy factored into positive Dehn twists. Conversely, given a supporting open book for a contact structure with monodromy factored into positive Dehn twists, one obtains a Weinstein filling.

One common question we ask is whether a single contact manifold has multiple Weinstein fillings. From the above construction, one possible way to attack this problem is to look for distinct positive factorizations of a given element in a mapping class group.

Theorem [Auroux]: There is an element in the mapping class group of the surface (of genus 1 and with one boundary component) with two distinct factorizations into positive Dehn twists such that the Weinstein fillings are distinguished by their first homology.

Remark: In this setting, the first homology is just given by where is the span of the vanishing cycles. The only real trick of Auroux is therefore to find a good candidate for the above theorem to hold, and just compute.

Generalizing a bit more:

Theorem [Baykur – Van Horn-Morris]: There exists an element in the mapping class group of (of genus 1 with three boundary components) which admits infinitely many positive factorizations such that the corresponding Weinstein fillings are all distinguished from each other by their first homology.

Finally, as one last application, I want to consider a result of Plamenvskaya and Van Horn-Morris, but I need to define the contact structures in question to begin. Honda’s classification of tight contact structures on the lens spaces can be formulated in Gompf’s surgery diagrams by the following diagrams, coming from a single 2-handle attachment to standard . We denote the corresponding contact structures by .

The surgery diagram for the contact structure .

Of these, the universal covers of and are also tight, where as the others’ universal covers are overtwisted. We say are virtually overtwisted.

Theorem [PV]: Each virtually overtwisted has a unique Weinstein filling (up to symplectic deformation) and a unique minimal weak filling.

Proof sketch: Let us first discuss the Weinstein part. There are a few nontrivial theorems which go into this, which we won’t discuss, but essentially we have the following sequence of results. The open book given by the surgery diagrams above induce open books with genus 0 pages. When we discussed Wendl’s theorem in part 2 of the J-holomorphic curve posts, one thing we mentioned was that one can apply his techniques when there is a planar open book (meaning pages have genus 0). He proves that if a contact manifold has a given supporting planar open book, then every Weinstein filling is diffeomorphic to one compatible with that specified planar open book. Hence, it suffices to study Lefschetz fibrations compatible with the one just described, which in turn becomes studying factorizations of an element in the mapping class group of , the disk with holes. A nontrivial result of Margalit and McCammond gives that every such presentation must be in a certain form, from which one can use smooth Kirby calculus to conclude that the surgery diagram must come from -surgery on some knot. Finally, an appeal to work of Kronheimer, Mrowka, Ozsváth, and Szabó using Seiberg-Witten Floer homology (also called monopole Floer homology) yields that this knot must have been an unknot, and since the framing is , this determines the canonical framing of the knot, which in turn implies we could only have had one of our original surgery diagrams.

Finally, to obtain the weak part, one can use work of Ohta and Ono to boost a weak filling up to a strong filling, from which Wendl’s theorem implies that any minimal weak filling is symplectic deformation equivalent to a Weinstein filling.

Kylerec – On J-holomorphic curves, part 2

This is a continuation post following part 1. Hope the delay wasn’t too long – more coming soon.

 

A preparatory comment on last time

Let us quickly recall the proof sketched in part 1 that fillable implies tight for contact . The idea was that if we had a filling , then the presence of an overtwisted disk locally gave a Bishop family of holomorphic disks as part of a 1-dimensional moduli space, but the compactified moduli space was seen to have only one boundary point. This was because continuing the family away from the center of the overtwisted disk could not lead to a possible boundary point – in our version, such a point would require a bubble, but we considered exact fillings.

It was mentioned last time that Eliashberg’s paper on fillings by holomorphic disks actually covers the weak case instead. The difference is that one now instead shows that any closed surface in a contact boundary which is weakly filled indeed bounds a 3-manifold which can be foliated by holomorphic disks. With this result, we never actually need to consider bubbles on the interior, so we can remove the exactness assumption.

I want to point this out because although this isn’t perfectly analagous to the discussion in the next section on Wendl’s paper, it is related in basic idea. The set-up is different, sure, and also one cannot work directly with the contact manifold with its filling (the necessity of a strong filling appears in the need to attach a positive cylindrical end), and finally also the completed foliation does have (isolated) nodal curves, but in the end we end up with a nice Lefschetz fibration with holomorphic fibers, and that’s pretty powerful, just like Eliashberg’s disk fillings. We explain this… now!

 

On Wendl’s Strongly fillable contact manifolds and J-holomorphic foliations

I should say, first of all, that one can generalize the results I am discussing here. For a somewhat more general discussion, see this post by Laura Starkston from 2013.

In this section, all contact manifolds are 3-dimensional and all symplectic manifolds are 4-dimensional.

We begin by recalling that the symplectization of a cooriented contact manifold is the symplectic manifold , where is the -coordinate. This symplectic manifold does not depend upon the choice of , since if we chose , then where is the diffeomorphism of sending .

Remark: One can define this in a more invariant way. The symplectization of is the set of covectors vanishing on . This is an -bundle over and is symplectic with respect to the standard symplectic form on . Fixing a local section over gives a coordinate for the fiber such that the symplectic form is just . We simply take the component where and coorients .

Given a symplectization, explicitly determined by a chosen contact form , one typically studies J-holomorphic curves only for choices of which are admissible, meaning:

• is -invariant
• 
• is a compatible almost complex structure for

Under these conditions, finite energy J-holomorphic curves from punctured Riemann surfaces are analytically easy to understand – the punctures are asymptotic to Reeb orbits at the positive and negative ends, and Gromov compactness extends to this setting in that one needs to include holomorphic buildings. One can imagine, for example a sequence of -holomorphic curves which look like some union of cylinders over Reeb orbits for all but a union of two intervals on which there is nontrivial behavior, where remains fixed but . In the limit, as these two intervals get farther apart, we break into two holomorphic curves in the symplectization. This forms what is sometimes called a holomorphic building. In general, there may be multiple levels in the limit, as in the figure below.

Here, a J-holomorphic curve gets stretched out in two places, shown in green, until eventually these green almost cylindrical parts get infinitely long. In the limit, we obtain a three story holomorphic building.

For more details in much more generality, one should consult this paper of Bourgeois, Eliashberg, Hofer, Wysocki, and Zehnder.

Now suppose that we have a strong filling of a contact manifold . Then by definition, we have a Liouville vector field whose flow allows me to identify a neighborhood of with a subset of the symplectization of the form where is a contact form for on . One can append the rest of the positive end of the symplectization, , to form a completed symplectic manifold . I can choose some compatible almost complex structure which far enough into the positive end is a restriction of some admissible . In this case, one can study J-holomorphic curves, and we have a similar Gromov compactness statement. In this case, our curves can either bubble, or form holomorphic buildings where the lowest level is just and whose higher levels are all .

Theorem (vaguely stated): Under some technical analytical conditions, an -invariant foliation of by -holomorphic curves of uniformly bounded energy will extend, with isolated nodal singularities, to the interior of (and hence to all of ).

Proof (sketch): We study the compactification of the moduli space of finite energy J-holomorphic curves in , and in particular, the closure of the component of the moduli space containing a special leaf in the symplectization end. This component is 2-dimensional, and hence is precisely given by the foliating leaves around it (recall is 4-dimensional). The closure of this component yields the full J-holomorphic foliation, where some isolated finite subset of the leaves are actually nodal curves.

In the end, by considering on which curve in the foliation a point is located, this yields a map , where the fibers are symplectic (since they are J-holomorphic and is compatible with ) and generically smooth except with finitely many nodal singular fibers, forming what is called a symplectic Lefschetz fibration.

We will discuss this notion more in a future post, where we will also see that Stein fillings correspond in some sense to certain (“allowable”) symplectic Lefschetz fibrations over a disk. Hence, one can ask – are there some examples of contact manfiolds on which we can find a finite energy foliation on the symplectization satisfying the correct analytical assumptions and such that ? The answer is yes in the case when is supported by a so-called planar open book, as was proved in this paper by Wendl. We will define this in a future post, but this discussion implies (up to how to tackle the word “allowable”) that:

Corollary: For contact 3-manifolds supported by a planar open book, strong and Stein fillability are equivalent.

Along similar lines, one can find finite energy foliations for the standard 3-torus (with contact structure induced by the restriction of the Liouville form on to the unit cotangent bundle). In this case, any strong filling, not just the standard one, would have , and so any strong filling of arises as the boundary of a Lefschetz fibration to . Wendl then beefs this up to prove, for example, that every minimal strong filling of is diffeomorphic to .

Finally, one can use these results to obstruct strong fillability in a manner analogous to the Bishop family argument. That is, if has a finite energy foliation satisfying the technical analytic assumptions, then one should be able to extend that foliation to a strong filling. Recall that the foliation extended by considering the component of the moduli space containing some specified leaf satisfying some conditions. If there is some other leaf which is not diffeomorphic to , then they cannot both be fibers of the same Lefschetz fibration, and so there couldn’t have been a strong filling in the first place. There are also other more technical versions of this argument, which for example allow one to reprove that positive Giroux torsion, i.e. that there is a contact embedding of , obstructs fillability, originally proved by David Gay using gauge-theoretic methods which are completely avoided in this approach.

 

On Barth-Geiges-Zehmisch’ The diffeomorphism type of fillings

As we have now seen twice, the technique of comparing moduli spaces of J-holomorphic curves to the topology of the situation in question is very powerful. We saw this both in our discussion last time of McDuff’s rational ruled classification, and we also just saw in our discussion of Wendl’s paper that the breaking which occurs in the compactification of a certain moduli space of curves in a strong filling of the positive end of a symplectization actually cooks up a Lefschetz fibration. One can view this paper as another instance of this way of thinking – here evaluation maps end up directly producing strong restrictions on the topology of a filling.

As we will see in a future post, Weinstein fillings of contact manifolds have a surgery theory consisting of handles of index at most , and so they have the homotopy type of a CW complex of at most this dimension. A subcritical Weinstein filling is then one where all the handles have index at most . The main theorem states that the existence of just one subcritical Weinstein filling places restrictions on the topology of any strong symplectically aspherical filling . By symplectically aspherical, we mean that .

Theorem [BGZ]: If is a contact manifold of dimension admitting a subcritical Stein filling with the homotopy type of a CW complex of dimension , then any strong symplectically aspherical filling satisfies

• for via the isomorphism induced by inclusion
• otherwise
• If , then all strong aspherical fillings of are diffeomorphic.

Corollary [Eliashberg-Floer-McDuff ’91]: Every symplectically aspherical filling of the standard contact sphere is diffeomorphic to a ball.

Remark: For , which is just the lens space , McDuff’s theorem from last time about fillings of lens spaces implies that there is a unique minimal filling up to diffeomorphism. By positivity of intersection, symplectically aspherical fillings are minimal, which implies the above result. But also, since is automatically a trivial cohomology class on the ball, McDuff’s result implies that the filling is in fact unique up to symplectomorphism. This result goes back to Gromov’s ’85 paper.

We won’t quite make it to a proof of the full theorem, but we will see some of the inner workings in the statement of the theorem stated below. We proceed by making an extra definition (not in Barth-Geiges-Zehmisch) to clarify the exposition.

Definition: Let  be a connected contact manifold and a Liouville manifold of finite type (meaning it is modelled after a positive symplectization outside of some compact region). Let be the corresponding Liouville vector field (satisfying ). The is called –spliffable (yes, this is what we called it at Kylerec) if is contactomorphic to a hypersurface in such that:

• is convex, meaning it is transverse to the vector field where is the standard radial Liouville vector field on
• the infinite component of is modelled after the positive symplectization of meaning this component is the union of the positive flow of along

Remark: A contact manifold which is fillable by a subcritical Weinstein manifold is spliffable. This follows from a result of Cieliebak that subcritical Stein manifolds are split.

Theorem: Let be an aspherical strong filling of a -spliffable contact manifold . Then there exists a commutative diagram of the form

(and similarly with replaced with ).

Remark: The Eliashberg-Floer-McDuff theorem is already a corollary of this weaker statement, using that is -spliffable, and using smooth topology.

Corollary: The unit cotangent bundle of a closed manifold admits no subcritical Weinstein fillings.

Proof: We need surjects onto with the standard unit disk filling and is -spliffable. But if admits a subcritical filling, then can be chosen to be subcritical so that . This is a contradiction.

Proof of the main theorem:

We begin by embedding into so that it is convex (which we can do by the spliffability condition). The interior component determine by the splitting through can then be replaced by by gluing in (since strong gluings are set up to be Liouville near the boundary). Call the interior of this manifold . We can then choose a map such that the interior embeds diffeomorphically onto . This embedding then gives us a smooth manifold which looks like but with the interior component replaced by . That is, .

We then wish to study some -holomorphic curves on this manifold. We pick a compatible which away from is of the form , where is admissible (as discussed in the previous section) for . We study the moduli space of -holomorphic spheres such that (for some large enough so that this slice misses ). We really want this up to reparametrization, so we fix slice conditions to define this moduli space: that , , and , for some choice of distinct and not .

The key about positive symplectization ends is that admissibility of the almost complex structure implies a maximum principle for these curves. This implies the following two items.

• Since looks like a symplectization, in , any curve in our moduli space must have actually just been .
• Any curve in our moduli space intersecting must intersect . First of all, is symplectically aspherical, so any holomorphic sphere must leave . But then, if it didn’t intersect , it would be contained completely in , which contradicts this maximum principle.

Now, this moduli space is an oriented manifold of dimension , and it comes with an evaluation map of the form . This map is actually proper and degree 1, which follows from the maximum principles just described, plus a little boost from positivity of intersection which implies that there is no need to worry about stable maps in the compactification of . This then restricts to a proper degree 1 evaluation map .

Hence, we obtain the following commutative diagram.

In homology, the right triangle becomes the desired triangle from the theorem.

As for the surjectivity part of the theorem, note that the leftmost vertical arrow is an isomorphism. Meanwhile, the bottom horizontal arrow is surjective for standard topological reasons (because one can cook up an explicit shriek map which is right inverse to on the level of homology).

 

Kylerec – On J-holomorphic curves, part 1

This to-be-2-part-because-this-got-long post is a continuation of the series on Kylerec 2017 starting with the previous post, and covers most of the talks from Days 2-3 of Kylerec, focusing on the use of J-holomorphic curves in the study of fillings. I should mention that two more sets of notes, by Orsola Capovilla-Searle and Cédric de Groote, have been uploaded to the website on this page. So if you wish to follow along, feel free to follow the notes there, and in particular, the relevant talks I’ll be discussing in this post are:

Part 1

• Day 1 Talk 1 – The introductory talk by (mostly) Roger Casals (with some words by Laura Starkston)
• Day 2 Talk 2 – Roberta Gaudagni’s talk introducing J-holomorphic curves
• Day 2 Talk 3 – Emily Maw’s talk on McDuff’s rational ruled classification

Part 2

• Day 2 Talk 4 – Agustín Moreno’s talk on Wendl’s J-holomorphic foliations
• Day 3 Talk 1 – Umut Varolgüneş’ talk on Barth-Geiges-Zehmisch’ diffeomorphism types of symplectic fillings

It should be obvious in what follows which parts of the exposition correspond to which talks, although what follows is perhaps a pretty biased account, with some parts amplified or added, and others skimmed or skipped.

 

J-holomorphic curves – basics

Gromov introduced the study of J-holomorphic curves into symplectic geometry in his famous 1985 paper, immediately revolutionizing the field. One might wonder why we care about these objects, and the rest of this post (along with part 2) should be a testament to some (but certainly not all) aspects of the power of the theory.

The “J” in “J-holomorphic” refers to some choice of almost complex structure on a manifold . Given an almost complex manifold, a J-holomorphic curve is a map such that is a Riemann surface and . In the case where is a complex manifold, we see this is precisely what it means to be holomorphic.

We are mostly concerned a choice of which is compatible with a symplectic manifold . By this, we mean that the (0,2)-tensor is a Riemannian metric. We say is tame if for each nonzero vector (note that as defined above is not necessarily symmetric in this case).

Proposition: The space of compatible almost complex structures on a symplectic manifold is non-empty and contractible. So is the space of tame almost complex structures.

This suggests either:

• Studying the space of J-holomorphic curves into for some particular choice of .
• Study some invariant of spaces of J-holomorphic curves which does not depend on the choice of compatible (or tame) with respect to a given symplectic form .

In walking down either of these paths, there are a large number of properties at our disposal. What is presented in this section is far from a conclusive list, and I have completely abandoned including proofs and motivation, so beware that there is a lot of subtlety involved in the analytic details. For many many many more details, consult this book of McDuff and Salamon.

Firstly, there is a dichotomy between somewhere injective curves and multiple covers. Some J-holomorphic curves will factor through branched covers, meaning that factors as such that the first map is a branched cover of Riemann surfaces. J-holomorphic curves which are not multiply covered are called simple, and it turns out that simple curves are characterized by being somewhere injective, meaning there is some for which and . Even better, somewhere injective means that is almost everywhere injective.

The main tool in the theory is the study of certain moduli spaces of J-holomorphic curves. There are many flavors of this, but we discuss a specific example to highlight the relevant aspects of the theory. The analytical details are typically easier for simple curves, so we denote by the moduli space of all simple -holomorphic curves. It turns out to be fruitful to focus in on a specific piece of this space, so we often restrict to a given domain of definition, say some , and also restrict the homology class of the map to some . The main question is:

When is such a moduli space actually a smooth manifold?

This is certainly a subtle question, and it turns out that not every works. However, it is a theorem that for generic , this moduli space is a smooth manifold of dimension , where .

Given our nice moduli space, we also might be interested in what happens as we change our choice of , so that we go from one regular choice to another. A generic path of such almost complex structures will give a smooth cobordism between the moduli spaces, a property which allows us to cook up invariants which do not depend, for example, on choices of compatible with a given symplectic structure.

To note a few variants of the discussion so far, sometimes we will study J-holomorphic disks with certain boundary conditions, or J-holomorphic curves with punctures sent to a certain asymptotic limit. In all cases, the same analytic machinery already swept under the rug (Fredholm theory) will give that the moduli spaces in question are smooth for generic choices of almost complex structure, and the dimension of this moduli space is given by some purely topological quantity (by, for example, the Atiyah-Singer index theorem).

One common thing to do is to quotient out by the group action given by reparametrizing the domain of a given J-holomorphic curve. That is, we consider the equivalence relation where is a biholomorphism. A more careful author would probably distinguish between the map as opposed to the corresponding equivalence class, which is really what one should mean when they say curve. Hence, one can quotient our moduli spaces by reparametrization to obtain moduli spaces of curves. Usually, these are the main objects of interest.

So now we have our nice moduli space, in whatever situation we desire, and we can ask about studying limits of J-holomorphic curves in that moduli space. In general, no such curve might exist. The first reason for this is that any such curve has an energy attached to it (when is compatible with ). If this quantity diverges to , then there can be no limiting curve. One can ask instead about what happens when the energy is bounded.

Consider the following sequence of holomorphic curves given by . We see that away from , this is just converging to the curve . But near , if we reparametrize the domain by , we see this converges to the sphere . In this case, our curve formed what is often called a bubble. More generally, a curve can split off many bubbles at a time. For an example of this, consider instead given by , in which a new bubble forms at in addition to the one discussed above. More generally, a sequence of curves can limit to a curve with trees of bubbles sticking out.

Such bubble trees are called stable or nodal or cusp curves (or probably a lot of other things), depending upon how old your reference is and to whom you talk. The incredible theorem, which goes under the name of Gromov compactness, is that this is the only phenomenon which precludes a limit from existing. We state this vaguely as follows:

Theorem [Gromov ’85]: The moduli space of curves of energy bounded by some constant (modulo reparametrization of domain) can be compactified by adding in stable curves of total energy bounded by .

Another generally important tool is that of the evaluation map. Suppose that we wish to study the moduli space of simple J-holomorphic maps in the homology class . Suppose is the group of biholomorphisms of . Then the group acts on by . Notice then that the evaluation map only depends on the orbit, and hence descends to a map . Proving enough properties of such an evaluation map sometimes allows us to compare the smooth topology of to that of . There are other variants of this – sometimes we wish to evaluate at multiple points, or sometimes we consider J-holomorphic discs and want to evaluate along boundary points. And often the evaluation map extends to the compactified moduli spaces considered above.

Finally, we come to dimension 4, where curves might actually generically intersect each other. With respect to these intersections, there are two key results to highlight. The first is positivity of intersection (due to Gromov and McDuff), which states that if any two J-holomorphic curves intersect, then the algebraic intersection number at each intersection point is positive (and precisely equal to 1 at transverse intersections). This can be thought of as some sort of rudimentary version of a so-called adjunction inequality (due to McDuff), which states that if is a simple J-holomorphic curve representing the class with geometric self-intersection number , then

.

Further, when is immersed and with transverse self-intersections, this is an equality, yielding an adjunction formula.

 

A first example – Fillable implies tight (in 3 dimensions)

On a first pass, I want to expand upon the example of fillability implying tightness in three dimensions which Roger Casals discussed in his introductory talk. Really, we prove the contrapositive – that an overtwisted contact manifold cannot be filled. For simplicity, we will consider exact fillings. This result is typically attributed to Gromov and Eliashberg, referencing Gromov’s ’85 paper as well as Eliashberg’s paper on filling by holomorphic discs from ’89. This is essentially the same proof in spirit, although we take a little bit of a cheat by considering exact fillings.

Firstly, recall that an overtwisted contact manifold is one such that there exists an embedding of a disk , such that the so-called characteristic foliation on , which is actually a singular foliation, looks like the following image, with one singular point in the center and a closed leaf as boundary.

So now suppose has an exact filling . We study the space of certain J-holomorphic disks with boundary on the overtwisted disk. The key is that a neighborhood of the overtwisted disk actually has a canonical neighborhood in up to symplectomorphism, and one can pick an almost complex structure to be in a standard form in this neighborhood. It turns out that with this standard choice, in a close enough neighborhood of the singular point in the interior of , all somewhere injective J-holomorphic curves are precisely those living in a 1-parameter family, called the Bishop family, which radiate outwards from the singular point .

Let us be a bit more precise, so that we can see this Bishop family explictly. Consider the standard 3-sphere , with its standard contact structure given by the complex tangencies, i.e. , with the standard complex structure on . Then consider the disk given by . The characteristic foliation on this disk looks like the characteristic foliation near the center of the overtwisted disk, so a neighborhood of this disk in yields a model for a neighborhood of the center of the overtwisted disk. We may assume the almost complex structure in this neighborhood is just given by the standard one, . Then the Bishop family is just the sequence of holomorphic disks given by for a real constant near 0. That these are all of the somewhere injective disks is a relatively easy exercise in analysis. Namely, suppose we had such a disk of the form . Then since boundary points are mapped to the overtwisted disk, . But each component of is harmonic, hence satisfies a maximum principle. Therefore, . But by holomorphicity, cannot have real rank 1 and so must be constant. Hence, any disk in consideration must have is a real constant.

All of these disks live in , but in particular in the slice where the second component is real, so we can draw this situation in by forgetting the imaginary part of . This is depicted in the following figure.

This Bishop family lives in some component of the moduli space of somewhere injective J-holomorphic disks with boundary on . Perturbing , one can assume this component is actually a smooth 1-dimensional manifold. We can compactify this moduli space by including stable maps, i.e. disks with bubbles, via Gromov compactness. On the Bishop family end, we see explicitly that the limit is just the constant disk at the point . So there must be another stable curve at the other boundary of this moduli space. We prove no such other stable curve can exist.

Similar to how we proved that the only disks completely contained in a neighborhood of the singular point on the overtwisted disk must have been part of the Bishop family, one can use a maximum principle argument to conclude that every holomorphic disk entering this neighborhood must have been in the Bishop family. Alternatively, one can use a modified version of positivity of intersections to conclude that continuing the moduli space away from the Bishop family, these boundaries have to continue radiating outward. Either way, the moduli space has to stay away from the central singularity of the overtwisted disk . But also, the boundary of a J-holomorphic disk cannot be tangent to , and in particular cannot be tangent to . This is by a maximum principle which comes from analytic convexity properties of a filled contact manifold.

The only possible explanation is that this is a stable curve with some sphere bubble having formed in the interior of . But one checks that the relation implies that for a -holomorphic sphere , we have . This vanishes by Stokes’ Theorem since is exact, and so must be constant, and so there is no bubble. In other words, this cannot explain the other boundary point of the component of the moduli space containing the Bishop family, so this yields a contradiction.

 

On McDuff’s The structure of ruled and rational symplectic 4-manifolds

Emily Maw’s talk from the workshop followed this paper by Dusa McDuff. In what follows, we shall consider triples such that is a smooth closed symplectic 4-manifold and is a rational curve, by which we mean a symplectically embedded . We call a rational curve  exceptional if with respect to the intersection product on (with respect to its orientation coming from ). We say is minimal if contains no exceptional curves. The main theorem is as follows:

Theorem [McDuff ’90]: If is minimal and , then is symplectomorphic to either:

• , in which case is either a complex line or a quadric (up to symplectomorphism).
• A symplectic -bundle over a compact manifold , in which case is either a fiber or a section (up to symplectomorphism).

Before describing the proof, which is the part involving J-holomorphic curve techniques, we apply this to strong fillings. We shall concern ourselves with fillings of the lens spaces with their standard contact structures, where is an integer. Let us first define this contact structure. Recall that the standard contact structure on is the one coming from complex tangencies by viewing . Then the standard contact structure on is the one given by the quotient where the action of given by preserves the contact structure, so that it descends.

Theorem [McDuff ’90]: The lens spaces all have minimal symplectic fillings , and when , these fillings are unique up to diffeomorphism, and further up to symplectomorphism upon fixing the cohomology class . The space has two nondiffeomorphic minimal fillings.

Proof (sketch): The complex line bundle over comes with a natural symplectic structure, and this forms a cap for . The zero section of is a rational curve of self intersection . McDuff’s explicit classification includes examples for any such given , and thus gives a minimal filling for . The remaining statements come from a more detailed analysis of the classification result.

Now, I will not go through all of the details of McDuff’s proof of the main theorem, but I will highlight where various J-holomorphic tools appear in the proof. Let me break up the proof into two big pieces.

Step 1: “Mega-Lemma” Consider minimal as above. There is a tame almost complex structure such that can be represented by a -holomorphic stable curve of the form , where:

• Each is -indecomposable (meaning any stable curve representing must actually be a legitimate curve of one component)
• The almost complex structure is regular for all curves in the class .
• The are distinct and embedded curves of self-intersection -1, 0, or 1, with at least one index for which .

We didn’t prove this at the workshop, so I won’t discuss it in detail here. But this is a major reduction into cases. For example, if and , then it had already been shown that this implies that . This bleeds into…

Step 2: Using the evaluation maps constructively

Let us discuss the proof of this last fact briefly. The idea is as follows. We consider the moduli space consisting of simple holomorphic spheres representing the class . This comes with an evaluation map of the form

where is the group of automorphisms of . Both sides have dimension 8 and this evaluation map is injective away from the diagonal since and we have positivity of intersection. Therefore, this map has degree 1, and so any pair of distinct points on has a unique curve passing through it. This is enough to show .

Let us do another case, but show that the adjunction formula also comes into play.

Proposition: Suppose is a simple homology class in (i.e. is not a multiple of another homology class) with , and suppose is a rational embedded sphere representing . Then there is a fibration with symplectic fibers and such that is one of the fibers.

Proof (sketch): The idea is to consider the moduli space of rational embedded -holomorphic curves with 1 marked point , and where is chosen to tame and such that is itself a -holomorphic curve, and where we have quotiented by reparametrization of the domain. Then one can compute the dimension of this moduli space at a given curve in the appropriate way as

,

where the last -4 comes from quotienting by the subgroup of fixing the marked point. Applying adjunction for the curve represented by , so that , yields . We also have an evaluation map

Since , there is at most one -curve through each point in , so it follows that this evaluation map has degree at most 1, and hence equal to 1 by regularity. This yields the structure of a fibration where the fibers are precisely the curves in our moduli space. Since the fibers are holomorphic, they are symplectic by the taming condition.

Kylerec Overview

Updates (June 11, 2017): Added link to other notes from Kylerec workshop, and fixed an error caught by Chris Wendl in the comments.

I’m very excited to be joining this blog!

This is the first of a series of posts about the content of the Kylerec workshop, held May 19-25 near Lake Tahoe, which focused on fillings of contact manifolds. Under the guidance of our mentors, Roger Casals, Steven Sivek, and Laura Starkston, we worked from the basic theory of fillings through some state-of-the-art results. Many of the basics have been discussed on this blog already in Laura Starkston’s posts from January 2013: Part 1 and Part 2 on Fillings of Contact Manifolds. For a more thorough introduction to types of filling and the differences between them, I suggest reading those posts (and the accompanying comments by Paolo Ghiginni and Chris Wendl). This post will remain self-contained anyway.

One can find notes that I took (except for three lectures, due to technical difficulties) at the Kylerec 2017 tab at this link. Other notes (with shorter load times, and including the ones I’m missing) will be posted on the Kylerec website soon are now posted on the Kylerec website here.

Comments and corrections are very welcome!

Definitions

We quickly review the various notions of fillings of a contact manifold. We shall always assume that our manifolds are oriented and contact structures cooriented. As a starting point, one might be interested in smooth fillings of contact manifolds. It turns out that this problem is rather uninteresting. Every contact manifold of dimension has a structure group which can be reduced to , but the complex bordism group is well known to satisfy . As a consequence, every contact manifold is smoothly fillable. We must therefore consider fillability questions which extend beyond the realm of complex bordism in order to discover interesting phenomena.

These notions are as follows, in (strictly!) increasing order of strength.

• We say a contact 3-manifold is weakly fillable if it is the smooth boundary of a symplectic manifold such that . There is a generalization in higher dimensions due to Massot, Niederkrüger, and Wendl, but we omit it here. (Simply requiring that is a positive symplectic form in the same conformal symplectic class as the natural one on , i.e. is up to scaling where is a contact form for , implies strong fillability in higher dimensions, by McDuff.)
• We say a contact manifold is strongly fillable if there is a weak filling such that one can find a Liouville vector field in a neighborhood of , i.e. one such that , such that gives a (properly cooriented) contact form for .
• We say a contact manifold is exactly fillable if there is a strong filling such that the Liouville vector field can be extended to all of . In other words, is the contact boundary of a Liouville domain where .
• We say a contact manifold is Weinstein (or Stein) fillable if it is exactly fillable by some , where , such that there is also a Morse function on such that is gradient-like for and is a maximal regular level set. In other words, is the contact boundary of a Weinstein domain.

As a final remark, there is a notion of overtwistedness in contact manifolds. In 3-dimensions, this is characterized by the existence of an overtwisted disk. This was known to obstruct all types of fillings, due to Eliashberg and Gromov. In higher dimensions, overtwistedness was defined in a paper of Borman, Eliashberg, and Murphy, which was discussed on this blog by Laura Starkston and Roger Casals, starting with this post and concluding with this one. This definition implies the existence of a plastikstufe as defined by Niederkrüger, which had been already shown to obstruct fillings (strongly in the same paper, weakly in the paper by Massot, Niederkrüger, and Wendl). In other words, in any dimension, overtwistedness implies not fillable. A contact manifold which is not overtwisted is called tight, so equivalently, fillable implies tight, in all dimensions.

To summarize this section:

Tight < Weakly fillable < Strongly fillable < Exactly fillable < Weinstein fillable

where all of the inclusions turn out to be strict.

Two Motivating Questions

Question 1: What tools do we have at each level of fillability?

The easiest type of filling to understand is that of the Weinstein filling, since Weinstein domains have an explicit surgery theory, which lends themselves to concrete geometric descriptions. Most notably, a Weinstein domain can be thought of as a symplectic Lefschetz fibration, which naturally has an open book decomposition on its boundary whose monodromy is a product of positive Dehn twists. Hence, Weinstein fillings and fillability can be studied through studying supporting open book decompositions for a contact manifold .

Another rather powerful tool is the study of J-holomorphic curves. Let us provide a quick example: the proof that fillability of a contact 3-manifold implies tightness. One assumes by way of contradiction that an overtwisted contact 3-manifold has a filling. Then one considers a certain compact 1-dimensional moduli space of J-holomorphic curves with boundary on the overtwisted disk. One finds an explicit component of this moduli space which has one endpoint (a constant disk) but cannot have another endpoint, which contradicts the compactness of the moduli space. In higher dimensions, studying similar moduli spaces of J-holomorphic curves yields obstructions to fillings.

There are some other miscellaneous techniques. For example, Liouville domains have attached to them a symplectic homology, which provides another tool for the case of exact fillings. And in the case of 3-dimensional contact manifolds, one can also study the Seiberg-Witten invariants of a given filling.

Question 2: How can we study the topology of different fillings? Or tell when fillings are distinct even if they have the same homology?

J-holomorphic curves come with extra evaluation maps which allow one to study how the moduli space of curves compares to some underlying topology, e.g. of the filling or of the contact manifold. This is a technique which comes up many times in different contexts, and it sometimes allows us to produce maps between the filling or the contact manifold in question which do not exist for any other obvious reason.

Similarly, symplectic homology in its two flavors and fits into an exact triangle with Morse homology, and so one can understand the topology of a filling from its symplectic homology. One might be interested, for example, in studying fillings with , in which case the homology of the filling is completely determined by . Alternatively, can be used directly to distinguish fillings.

Overview of Kylerec

More detailed posts about the contents of Kylerec will appear in future blog posts, but I will outline here precisely what was covered.

Day 1: After an overview talk, we spent the rest of the day studying the surgery theory of Weinstein manifolds, and began our study of the correspondence between Weinstein fillings, Lefschetz fibrations, and open book decompositions.

Day 2: We highlighted some results from this correspondence, and then turned towards an introduction to the theory of J-holomorphic curves, including applications of this theory to fillings via McDuff’s classification result as well as Wendl’s J-holomorphic foliations.

Day 3: On our short day, we first discussed some applications of J-holomorphic curves to high-dimensional fillings due to Barth, Geiges, and Zehmisch (for example reproving the result of Eliashberg, Floer, and McDuff that the standard sphere has a unique aspherical filling), and applied Wendl’s theorem (as discussed in Day 2) following a paper of Plamenevskaya and Van Horn-Morris to show that many contact structures on the lens spaces have unique Weinstein fillings up to deformation equivalence.

Day 4: We discussed the Seiberg-Witten equations, how they appear in symplectic geometry, and how they are used by Lisca and Matic to distinguish contact structures on homology 3-spheres which are homotopic (through plane fields) but not isotopic (through contact structures). We also discussed how Calabi-Yau caps, as defined by Li, Mak, and Yasui, can be used to prove certain uniqueness results on fillings of unit cotangent bundles of surfaces, as in this paper by Sivek and Van Horn-Morris.

Day 5: On our last day, we focused mainly on symplectic homology (and its variants). In one talk, we performed computations which allowed us to distinguish contact structures on standard spheres (see Ustilovsky’s paper) and to compute the symplectic homology of fillings of certain Brieskorn spheres (see Uebele’s paper). We also discussed Lazarev’s generalization of M.-L.Yau’s theorem (that subcritical Weinstein fillings have isomorphic integral cohomology) to the flexible case.